Arts and Science: Counting Out Loud

MONDAY, 3 FEBRUARY 2014

To many of us, the idea of a number is inseparable from its representation in language. It is difficult to think of the abstract concept of ‘two’, without calling to mind the actual word two. Our overarching sense of what two is includes both its numerical definition and the means by which this can be expressed to others. However, the connection between language and mathematics shows considerable variation between languages – perhaps indicative that the etymology of numbers is sometimes more arbitrary than it is organic.

One of the fundamental principles that most languages are built upon is that of the base 10 or decimal system, a system for dealing with numbers attributed to our having 10 fingers and thumbs with which to count. In English words for the numbers 11-19, with ‘teen’, an older form of ten, being added to three, four, five, and so on. The oddities in this list, eleven and twelve, can be traced to similar origins in the old German words ein-lif and zwo-lif, lif meaning ten. Similarly, the words for twenty, thirty, forty and so on are derived from contractions of ‘two tens’, ‘three tens’ and ‘four tens’.

However, the decimal system is far from universal. The most widespread alternative is that of the vigesimal, or base 20. Languages that use this system appear all around the world, from Mayan to Danish. As vigesimal is most likely based on counting with hands and feet, its adoption by diverse civilizations is not a surprise. Interestingly enough, the implementation of the base 20 system often comes with certain localised quirks and exceptions depending on the language. In the traditional form of Welsh for example, despite being a vigesimal system, 15 (‘pymtheg’) acts as a pivot number, with 16 being ‘un ar bymtheg’, literally ‘one on fifteen’. A similar scheme holds for 17 and 19 (‘two on fifteen’ and ‘four on fifteen’ respectively), but strangely enough 18 introduces a further exception, being ‘deunaw’ (‘two nines’) rather than ‘three on fifteen’.

Sometimes these differences can exist even in the same base language. The classic example of this case is French, and its confusing mixture of decimal and vigesimal systems. In modern official French, the numbers up to 69 are based on decimal system. Sixty, for example, is ‘soixante’ (meaning six tens). After this point however they switch to a vigesimal system for 70 to 99 – seventy is ‘soixante- dix’ or sixty-ten, and eighty is ‘quatre-vingts’ or four-twenties. The mixture is most probably due to an attempt to standardise to a decimal system from a formerly vigesimal one, which some scholars claim comes from French’s link with older Celtic languages, such as Welsh.

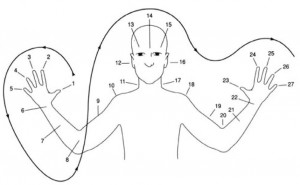
While the two dominant systems present around the world happened to evolve (most probably) from the fact we have 10 digits on our hands and feet, this doesn’t mean that systems based on other physical attributes do not exist. An extreme example comes from the Oksapmin people of New Guinea, who use a base 27 counting system, derived from the names of the 27 different body parts they use for counting. They start with ‘tip^na’, or thumb, for one, going along their arm (six is ‘dopa’, or wrist), to their head (twelve is ‘nata’, or ear), before going down the other side (sixteen is ‘tan-nata’, or ear on the other side), ending with ‘tan-h^th^ta’, or pinky on the other side, for twenty seven. Whilst most refer to mathematics and science when they speak of the wonders of numbers, truly there is much complexity and beauty present in the language we use to express our numeric ideas.

Matthew Dunstan is a 3rd year PhD student at the Department of Chemistry